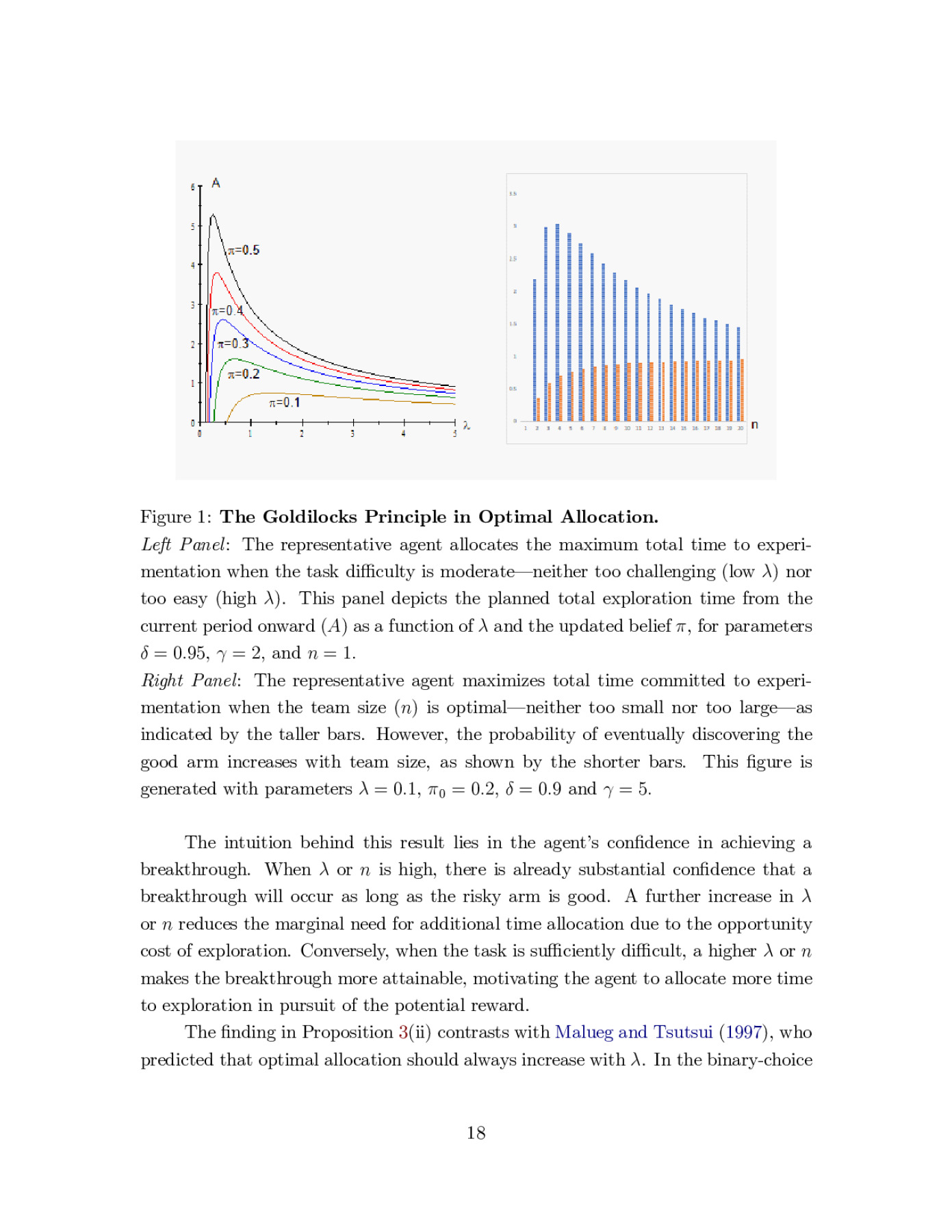
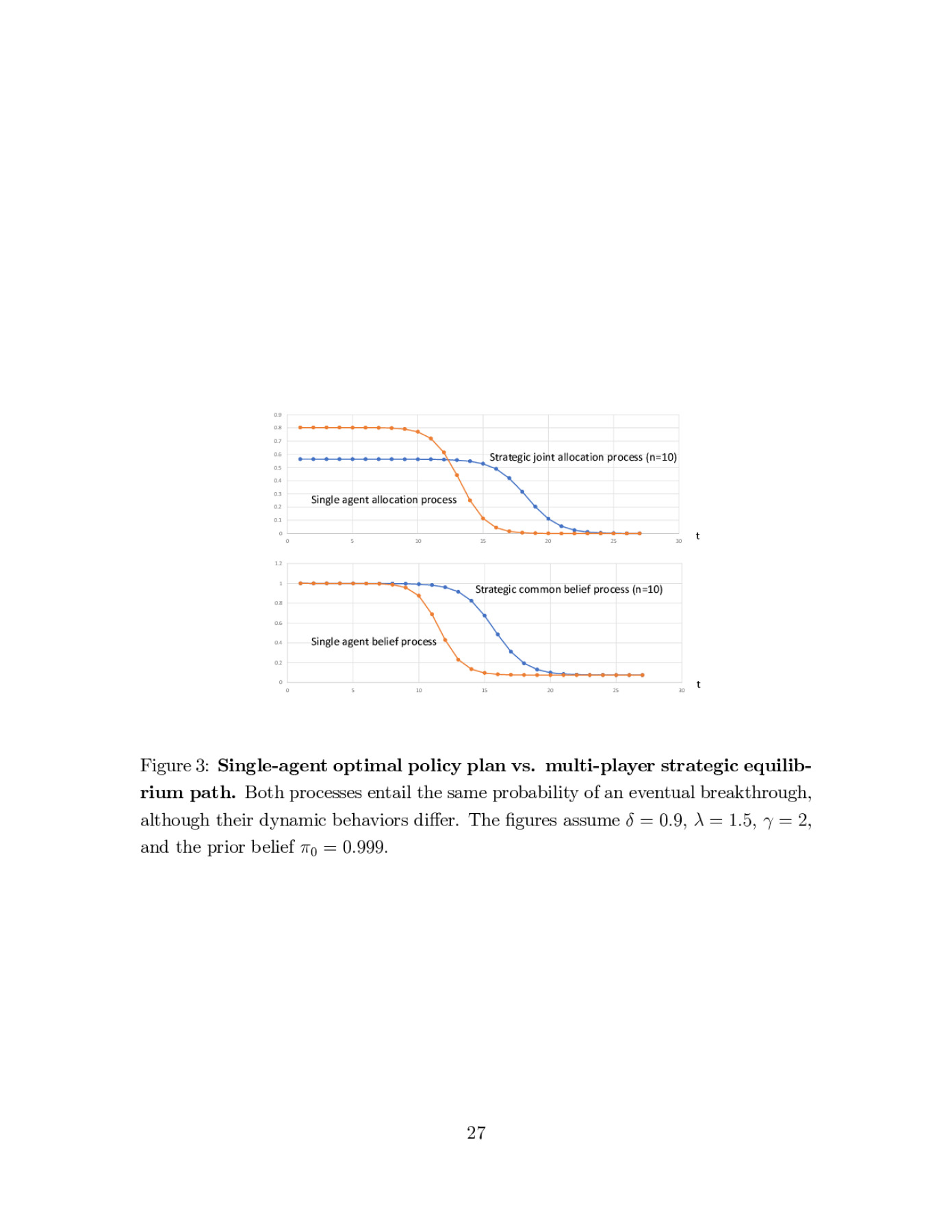
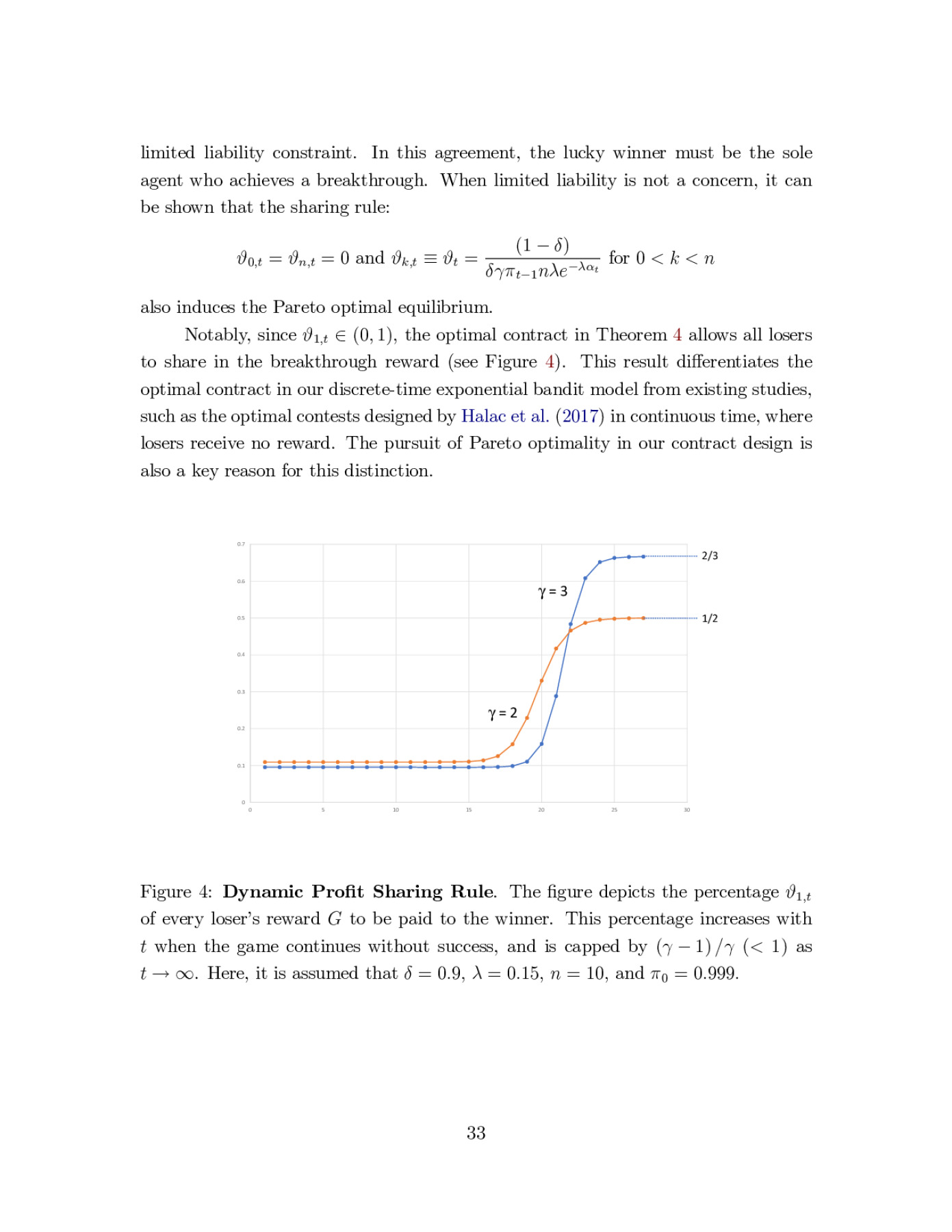
2024 Australasia Meeting, Melbourne, Australia: December, 2024
Efficient Resource Allocation in Discrete-Time Breakthrough Bandit Models
Audrey Hu and Liang Zou
We study a two-armed bandit model in discrete time, where a team of agents allocates limited resources (e.g., time) per period to achieve a breakthrough under uncertainty. In the cooperative case, we characterize (Pareto) efficient allocation strategies with interior solutions, improving upon the binary strategies prevalent in existing literature. These strategies exhibit two notable features: (i) persistence, where experimentation continues until a breakthrough is achieved, and (ii) adherence to a "Goldilocks principle," whereby each agent's incentives to experiment are maximized at specific team sizes or task difficulties. In the noncooperative case, we identify a symmetric equilibrium of strategic experimentation, characterized by free-riding, inefficiency, and procrastination, with no other equilibria existing. We further demonstrate that Pareto-efficient outcomes can be restored in equilibrium using a dynamic profit-sharing contract that satisfies budget-balancing and limited-liability constraints.
Preview